Research Labworks for MSc
The Research Labwork in Physics in the 1. and 2. master semester serves the purpose of training in a specific physical issue as well as project planning in order to improve experimental skills.
One project should be completed during the semester at one afternoon per week (4 CP). The experiments can be chosen from one of the following topics: optics, solid state physics, astronomy, computational physics, and material science. Depending on the specific conditions, one project can be done by either on or more students, in the latter case, with complementary tasks.
Students who would like to take the Research Labwork in the summer semester 2025 please register immediately with the advanced lab course office or in Friedolin. The registration for the respective projects in the moodle list in this summer semester will start Wednesday, March 19th, 2025 at 10 am.
At the end of the summer semester students submit (at the very latest on 15.08.2025) their results in form of a scientific paper draft.
The Organization of the research labwork is managed by the F-Praktikum office. If there are any questions, please contact us via physik.f-praktikum@uni-jena.de. Please do not send requests individually to project supervisors.
-
Advanced Experimental Microscopy - Super-Resolution Microscopy
Seeing is believing. This sentence is as true as it is tricky. Most cellular components and processes, crucial for the nuanced understanding of (human) life, are not observable by conventional light microscopy since Abbe’s Law describes their maximum resolution to roughly half the wavelength of the observed light. This law is literally set in stone in Jena. However, over the past 15 years several ways of cleverly circumventing this diffraction limit were developed and implemented, achieving three-dimensional resolutions down to the nanometer range, resulting in the ever-growing field of optical super-resolution microscopy, for which the 2014 Nobel Prize in Chemistry was awarded.
The aim of this projects is to introduce, understand and apply the principles of state of the art fluorescence microscopy techniques, used e.g. in a broad range of modern biomedical and cell-biological research. Students prepare their own, fluorescently labeled, biological samples and will image them on a variety of advanced microscopes with different (resolution) capabilities. The qualitative and quantitative comparison of acquired images will illustrate the advantages and limitations of the respective microscopy technique.
Goals and Context
- Principles and application of advanced fluorescence microscopy techniques
- Concept of diffraction-limited and super-resolution
- Preparation of fluorescently labeled, biological samples
- 3D & multi-colour imaging at the nanoscale
Methods
- Cell culture and wet lab
- Fluorescent labeling
- A selection of advanced fluorescence microscopy techniques from the IAOB toolbox:
- Confocal Laser Scanning Microscopy
- Array Scan Microscopy
- Stimulated Emission Depletion (STED)
- Structured Illumination Microscopy (SIM)
- Single-Molecule Localization Microscopy (SMLM)
- MINFLUX Nanoscopy
- Image analysis by Fiji/ImageJ
Prerequisites
- An open mind and motivation for independent thinking
- Students should be able to explain the general difference between confocal and widefield microscopy and have basic knowledge on the concepts of super-resolution microscopy (e.g. Abbe’s diffraction limit)
- You should know the basic principles of fluorescence
A good preparation for the course is the biophysics lecture from Prof. C. Eggeling
Person in charge: Christian Franke & Katharina Reglinski
Supervisors: Christian Franke & Katharina Reglinski
Venue: Microscopy Labs of the IOAB in the ZAF and Abbeanum or at the IPHT (Beutenberg)
The topic is suitable for two groups with 1-2 students each.
-
Advanced techniques for stabilization of optical cavities
Advanced measurement techniques and stable optical systems are crucial for scientific research
breakthroughs in fields like quantum optics, spectroscopy, and fundamental physics. These
techniques enable precise probing of matter and light properties, as well as detection of phenomena like gravitational waves. At the core are stabilization methods for optical cavities and lasers. Optical cavities enhance light-matter interactions for high-precision measurements. Cavity stabilization ensures reliability and precise control over photon generation and manipulation. The presentation will cover general principles and techniques for stabilizing optical systems, beyond specific applications. It will explore advanced stabilization methods like Side-of-Fringe (SOF) locking and Pound-Drever-Hall (PDH) locking, which have wide applicability in diverse scientific settings.Teaching Goals and Content
- Understand the principles and importance of optical cavities in current technologies.
- Design and construction of an optical cavity.
- Calculations of the mode-matching optics of a cavity by using ABCD matrix.
- Explore the Side-of-Fringe (SOF) locking technique for cavity stabilization.
- Explore the Pound-Drever-Hall (PDH) locking technique for stabilizing optical cavities by using radiofrequency techniques.
- Compare and contrast the SOF and PDH locking techniques in terms of performance and applicability.
- Analyze the stability and reliability of the optical cavity using these locking techniques.
Experimental Techniques and Equipment
- Optical alignment of optical cavities.
- Continuous wave pump lasers at suitable wavelengths.
- Photodetectors for monitoring the cavity's reflected and transmitted light.
- Electro-optic modulators for phase modulation in the PDH technique.
- Lock-in amplifiers for demodulation and proportional-integral (PID systems) for feedback control.
- Data acquisition systems for recording and analyzing the locking signals.
Contact:
Place: Fraunhofer IOF institute
Supervision: MSc. L. Gonzalez
For this experiment two students are recommended.
-
Dispersion Characterization of fs-written chirped fiber Bragg gratings
Fiber Bragg gratings (FBG) play a key role in modern fiber optics. They consist of a modulated refractive index in the fiber core and act as narrow-bandwidth filters or in-fiber reflectors. Their application spans from cavity mirrors or dispersion control in fiber lasers to signal filtering in quantum optics and astro photonics. They are also commonly used as strain or temperature sensors. In comparison to normal FBGs, that have a constant grating period, chirped FBGs have a varying(increasing) grating period, meaning different spectral components will be reflected at different spatial positions within the grating. This enables dispersion control in the fiber. To realize FBGs, a femtosecond pulsed laser system is used.
The main goal of this project is to automate an existing dispersion measurement setup. To achieve this, a linear stage will be integrated into the simple in-fiber interferometric measurement system and subsequently characterized. This stage, along with a sweeping laser source, will then be controlled automatically via software. Afterward, the dispersion of several FBGs will be measured using in-house software. Finally, the results will be analyzed and compared.
Teaching goals and content
- Basics of FBGs and chirped FBGs and their inscription by femtosecond pulsed laser
- Fiber handling and preparation
- Setup and characterization of a simple interferometric fiber-based setup
- Measurement of dispersion of multiple different FBGs
- Basic signal processing and analysis
- Automation processes
Prerequisites
- Basics in optics,
- Minimal experimental skills
- Basic knowledge in programming, ideally in Python\Matlab\LabView
- Interest in integrated fiber optics and their application
Contact:
Supervisor: Georg Schwartz
Place: Institute of Applied Physics (IAP, Beutenberg)
This experiment can be carried out by one of two students.
-
Femtosecond Laser
Nowadays the generation of ultra-short laser pulses with a duration down to some femto seconds is state of the art. Such pulses find their application not only in the field of scientific research to investigate ultra-fast processes, to perform ultra-precise spectroscopy, or to generate extreme electrical and magnetic fields through ultra-high light intensities, but they are also applied in material processing, medicine, especially in ophthalmology. Nevertheless, the generation and metrology of ultra-short pulses require complex measurement techniques. The basics to understand the underlying effects of pulse generation, stretching and compression as well as their measurement will be taught here. Some of these effects are based on non-linear optics and frequency conversion, that requires phase matching to get reasonable efficiencies. Second harmonic generation and two-photon absorption are used for pulse characterization by auto-correlation here. The limitations of the auto-correlation for the reconstruction of the temporal behavior of the laser field will be investigated in more detail.
Teaching goals and content
- Working principle and properties of solid-state lasers (Ti:sapphire)
- Cavity stability and longitudinal cavity modes
- Dependence of output power on pump power
- Generation of femtosecond pulses by Kerr-lens mode-locking
- Compensation of group velocity dispersion in optical cavities
- Impact of spectral phase on pulse duration and temporal pulse shape
- Measurement of band-width and duration of laser pulses
- Application of Fourier-Transform to explain pulse stretching and compression
- Interferometric and intensity auto-correlation and their limitations for pulse characterization
- Measurement of group velocity dispersion (GVD) of several materials
Experimental techniques and equipment
- diode-pumped, frequency-doubled 5W Nd:YV04-laser as pump source
- homemade Ti:sapphire femtosecond laser with prism GVD compensation
- external prism pulse compressor
- optical spectrometer
- second harmonic generating auto-correlator
- photodiodes, powermeter and oscilloscope
Contact:
Supervisor: Dr. Joachim Hein
Place: F-Praktikum
For this experiment two students are recommended.
-
Femtosecond pulse chirp estimation
Ultrashort laser pulses have a large spectral width. These pulses acquire a certain amount of chirp (modulation of the spectral phase) and thus change their temporal shape when propagating in a material with dispersion. Measuring the pulse spectrum is not sufficient to estimate the pulse width and shape because it does not contain information about the spectral phase of the pulse. Although there are some sophisticated measurement techniques available to reveal this, a simpler approach is pursued here: the extraction of spectral phase information from a second order interferometric autocorrelation. Such an autocorrelator is used to measure the pulse width of a home-built femtosecond Ti:sapphire laser. When the pulses contain a significant amount of chirp, pulse width estimation is not as simple as extracting it from the width of the autocorrelation trace. The goal of this project is to write a program, e.g. in Phython, Julia or any other programming language, to compute the higher order chirp coefficients from the measured spectrum and the interferometric autocorrelation trace. This code could be used to automate the temporal pulse characterization.
Teaching goals and content
- Working principle of second harmonic autocorrelation
- Behavior of femtosecond pulses with chirp
- Application of programming tools for data evaluation
- Programming with open source software
Experimental techniques and equipment
- homemade Ti:sapphire femtosecond laser with prism GVD compensation
- optical spectrometer
- second harmonic generating auto-correlator
- photodiodes, powermeter and oscilloscope
Contact:
Supervisor: Dr. J. Hein
Place: F-Praktikum
For this computational project one student is recommended, but two are possible as well.
-
High Resolution Computational Imaging – Ptychography
Ptychography is an advanced computational imaging technique used in electron, X-ray, EUV, and visible-light microscopy to achieve high-resolution images beyond the limits of conventional lenses. It is a form of coherent diffraction imaging (CDI) that reconstructs an object's structure by analysing diffraction patterns from multiple overlapping illumination positions. In particular, it enables quantitative imaging of the sample’s transmission function in both amplitude and phase, providing access to the local density, local material composition, and local thickness of complex structures.
The aim of this project is to apply the ptychography technique to imaging of vaious biological samples and understand is principles and advantages. You will particularly investigate the influence of different scan grids and illumination beams on the image quality and resolution.
Teaching goals and content
- Understand the basics of ptychography
- Perform systematic ptychography measurements on various samples
- Apply the pty:lab toolbox to reconstruct Ptychography datasets
- Explore the influence of the scan grid and different illumination beams on image quality and resolution
Experimental techniques and equipment
- Visible ligth ptychography setup
- Pty:Lab computational toolbox (https://github.com/PtyLabExterner Link)
- Different laser sources
- Scientific CMOS detectors
Prerequisites
- Interest in computational imaging
- Basics knowledge in Optics and Fourier-Optics
- Basic programming skills in Python or Matlab
- Experimental skills (optical setups) and problem-solving ability
Contact:
Supervisor: Jan Rothhardt and Cesar Jauregui
Place: Institute of Applied Physics, Abbe-Center-of-Phtonics (Beutenberg)
The topic is suitable for one or two students.
-
Imaging without imaging: using algorithms to replace optics
In modern optical imaging, precise methods for investigating micro- and nanoscale structures are of great importance. Diffraction imaging revolutionizes optical imaging by foregoing traditional optics and instead relying on computer algorithms to create high-resolution images. Despite its potential, there are significant challenges in reconstructing images from diffraction patterns.
The main focus of this project is to investigate the optical diffraction imaging in the visible range. The various influences such as the size of the illumination spot, the coherence, the structure size, the monochromaticity or bandwidth and the overlap with other beams in the visible spectral range will be taken into account. A particular focus will be on exploring the convergence of reconstruction algorithms as a function of the above parameters. In particular, multicolor diffraction still raises many fundamental questions.
Teaching Goals and Content
- Design and construction of an optical test setup
- Basics of diffraction imaging and ptychography
- Influence of various light sources on imaging
- Development and application of reconstruction algorithms
- Experimental applications and diagnostic methods
Prerequisites
- Basics in optics, Fourier optics, and image processing
- Interest in modern imaging and algorithm development
- Experimental skill and problem-solving ability
- Basic knowledge in programming, ideally in Python or Matlab
Contact:
Supervisor: Dr. Martin Wünsche and Dr. Jan Rothhardt
Place: Max-Wien-Platz 1 and Albert-Einstein-Str. 6
The topic is suitable for two groups with 2 students each.
-
Stimulated amplification of high-order harmonics in semiconductor crystals
The goal of the suggested project is to investigate experimentally the possibility to combine within the same volume of a semiconductor crystal the conventional laser amplification and extreme nonlinear process of high-order harmonic generation. The experiment is based on a pump-probe scheme where an ultrashort pump pulse creates the population inversion in the volume of a ZnO crystal via resonant or multi-photon absorption. This population inversion is probed by a high-order harmonic, resonant with the amplification band and generated with a controllable delay in the same crystal volume by an ultrashort mid-IR pulse. The goal is to realize the stimulated amplification of the harmonic and to investigate the spectral shape of the gain and its time-resolved dynamics as a function of pump intensity, seeding harmonic intensity and mutual polarization of pump and probe. These results will lay a foundation for the realization of ZnO nanowire lasers generating ultrashort (femtosecond) laser pulses.
Prerequisites:
- Basics knowledge in optics
- Good experimental skills
Contact:
Supervisor: Dr. Daniil Kartashov
Place: Institute of Optics and Quantum Electronics (IOQ, Max-Wien-Platz 1)
The topic is suitable for one or two students.
-
Ultrafast fiber laser oscillators
Ultrashort pulse lasers are nowadays one of the most interesting types of lasers, since they have opened up new applications in the scientific, medical and industrial fields. In fact, achieving ultrashort pulses (<1ps) is a unique ability of lasers that separate them from other light sources. Usually such ultrashort pulses, which are some of the shortest events ever created by Mankind, are obtained using the technique of mode locking, which has become one of the most important methods in modern lasers.
Additionally, among all available laser architectures, fiber lasers have stablished themselves as one of the most attractive types of lasers due to their simplicity, efficiency, low-cost, maintenance-free nature, compactness, robustness and high-power scalability. In fact, fiber lasers are currently replacing more traditional types of lasers in many applications.
In this project, you will get to know fiber lasers by building and characterizing a mode-locked fiber laser able to deliver several 100 fs pulses. In this project you will build the cavity, try out different configurations and learn about the physics of mode locking. At the end, you will have created from scratch one of the most appealing types of lasers: an ultrafast fiber laser.
Teaching Goals and Content
- Understand the principles of mode-locking and fiber lasers.
- Design and construct a fiber cavity.
- Use of Semiconductor-Saturable Absorber Mirrors (SESAMs) to achieve mode-locking.
- Learn to characterize an ultrafast laser.
- Analyze the performance of the laser as different parameters of the cavity are changed.
- Perform simulations of the laser.
Experimental Techniques and Equipment
- Handling of optical fibers (stripping, cleaving, splicing, etc).
- Coupling of optical radiation in/out of a fiber.
- Use of SESAMs.
- Systematic characterization of the laser performance using, e.g. power meters, spectrometers, etc.
Contact:
Supervisor: Cesar Jauregui & Jan Rothhardt
Place: Institute of Applied Physics/Abbe center of Photonics
This experiment can be carried out by one of two students.
-
Exfoliation and Optical Characterization of 2D materials
The realization of the first monolayer graphene flake in 2004 has triggered a vast amount of experimental and theoretical research, leading also, among other results, to the discovery and fabrication of several other atomically thin materials, such as the semiconducting transition metal dichalcogenides (TMDs). Besides the interest for fundamental science, 2D materials play an important role from the technological point of view and will likely contribute to develop the next generation of electronic, optoelectronic, and energy storage devices.
In this series of experiments, graphite and TMDs bulk crystals will be exfoliated and transferred onto a silicon/silicon dioxide substrate and characterized by optical spectroscopy methods. The students, after successful mechanical exfoliation and transfer, will learn how to identify samples with different number of layers using the optical contrast method. Subsequently, they will proceed to further characterization by Raman and photoluminescence (PL) spectroscopy. Finally, students will fabricate layered heterostructures (TMD/graphene) and they will perform power-dependent PL measurements to study electron interactions and interlayer charge transfer.
Thus, the tentative working plan includes the following lectures and experiments:
Weeks 1-3
- Introduction to TMDs: band structure, optical properties, fabrication methods
- Theory: identification of number of layers in TMDs from optical contrast, Raman and PL spectroscopy
- Exfoliation of TMD crystals and identification of the number of layers by optical contrast and PL spectroscopy
Weeks 4-6
- Introduction to graphene: band structure, optical properties, fabrication methods
- Theory: identification of number of layers in graphene from optical contrast and Raman spectroscopy
- Exfoliation of graphite crystals and identification of the number of layers by optical contrast and Raman spectroscopy
Weeks 7-10
- Fabrication of a layered heterostructures (TMD/graphene)
- Power-dependent PL measurements of monolayer and heterobilayer samples
Weeks 11-14
- Data analysis and discussion
- Report writing
Objectives
- Graphene and TMDs: fabrication and optical properties
- Laser: basics of laser science
- Optical characterization: optical contrast, Raman and PL spectroscopy
Experimental techniques
- Mechanical exfoliation and deterministic transfer of graphene and TMDs
- Optical contrast, Raman and PL
Contact:
Supervisor: Muhammad Hussain
Venue: GUFOS, IFK (Room E012)
The topic can be worked on by one or two students. Supervision is possible in English only.
-
In-situ Flux Measurement in a Broad Ion Source
Ion sources are applied in a wide range of processes for e.g. doping, quantum dot fabrication and the creation of buried layers. For theses purposes, broad beam ion sources can be utilized to maintain fast processing times even on large implantation areas.
In 2022, operation of a unique four-grid accelerator broad ion source (4GABIS) started at the IAP Jena. Within 4GABIS, ions are accelerated from a plasma source with voltages of up to 30 kV in a beam of around 180 mm diameter. Currently, 4GABIS is not completely characterized for all of the available acceleration parameters. Hence, the first goal of the experiments is to investigate the effect of grid voltages on the resulting shape of the beam profile. This will be measured both directly with a movable faraday cup and indirectly via resulting sputter rates. The insights of this will subsequently be applied to establish ratios between the flux of ions hitting the target and the faraday cup in measurement position, respectively. Next to that, the impact of neutralisation of ions during their flight via charge transfer will be measured. From this, charge transfer cross sections can be calculated and compared with the literature. The combined results are to be integrated into a pre-existing LabVIEW program to allow for in-situ measurement of ion flux.
Summary of the main goals for this experiment:
- Understanding the shaping of a hot-cathode glow discharge plasma
- Investigation of grid voltage parameters to affect the resulting ion beam
- Measurement of charge transfer cross section for collisions of ions and residual gas
- Combining parameters necessary for in-situ monitoring of ion flux
Prerequisites:
- Basic understanding of hot cathode glow discharge and ion acceleration
- Basic knowledge of LabVIEW programming
- Good laboratory skills
- Interest in operating a unique ion source
Contact:
Supervisor: Johannes Kaufmann
Venue: Institute of Applied Physics, Beutenberg Campus (Albert-Einstein-Str. 15)
The topic is suitable for one or two students.
-
Investigation of Multilayer Mirrors for X-Rays
Learning target and topics
- Thin metal layers: deposition, characterization of the layer properties and structure
- High vacuum technology
- Introduction and application of various analysis methods
- Scanning Electron Microscopy (SEM) and Energy Dispersive X-Ray Analysis (EDX)
- Scanning Tunneling or Atomic Force Microscopy (STM, AFM)
- AugerElectronSpectroscopy (AES)
- X-ray diffractometry
Experimental equipment
- Sputter coating system from Oxford Instruments
- Thermal evaporation system (self-made)
- Mass Spectrometer for residual gas analysis
- Scanning Electron Microscope
- Atomic Force Microscope
- Scanning Tunneling Microscope
- Auger Electron Spectrometer
Supervisor: Dr. Thomas Siefke, Dr. Berit Marx-Glowna
Venue: F-Praktikum, IFK and IOQ
The topic is suitable for one or two students.
-
Low-Cost Single Crystalline Metal Layers: Fabrication and Characterization
Single crystalline metal layers on natural mica are often used in electronic devices such as transistors, solar cells, and sensors, due to their high electrical conductivity and mechanical stability. Additionally, they can be used as a substrate for growing other single crystalline materials, such as semiconductors, which can be used in electronic devices as well. The high thermal and chemical stability of natural mica also makes it an ideal substrate for a wide range of applications, such as in the aerospace and automotive industries. Overall, the cost-effective fabrication of single crystalline metal layers on natural mica can have a significant impact on the development of new technologies and the improvement of existing ones.
The experiment aims to fabricate and investigate the properties of single crystalline metal layers using a thermal evaporation method. In this process, the metal material will be thermally evaporated onto a substrate of natural mica under specific conditions, such as temperature, pressure, and evaporation rate, to achieve single crystalline growth. The substrate will be carefully chosen, cleaned and prepared to ensure optimal growth conditions.
The characterization of the fabricated metal layers will be done using a combination of techniques including atomic force microscopy (AFM) and x-ray diffraction studies (XRD). The AFM will be used to observe the surface morphology of the metal layers, including the thickness, uniformity, and surface roughness. The XRD will be used to determine the crystal structure of the metal layers, including the crystal size, lattice spacing, and crystal orientation, as well as to identify any defects or impurities in the crystal structure.
The goal of the experiment is to understand how the thermal evaporation fabrication method and process conditions affect the properties of single crystalline metal layers and how such layers can be used in various applications such as electronics, catalysis, and sensing. The experiment will also help in understanding the relationship between the growth conditions and the crystal structure and will provide a better understanding of the fundamental physics of metal growth.
The main goals of this experiment are:
- Fabrication of single crystalline metal layers using thermal evaporation
- Investigation of the structural and morphological properties
Prerequisites:
- Familiarity with basic laboratory techniques
- Basic understanding of crystal growth and crystal structure
Methods:
- Thermal evaporation setup
- Atomic force microscopy (AFM)
- X-ray diffraction studies (XRD)
Contact:
Supervisor: Dr. Marco Grünewald and Dr. Berit Marx
Language: German or English
Venue: F-Praktikum and labs of the IOQ
The topic is suitable for one or two students.
-
NanoFabLab
Micro- and nanotechnology forms the basis for a growing number of everyday objects and current scientific research. Many physical systems require a direct examination or at least a basic understanding of this technology chain.
The theoretical foundations are already taught at the FSU as part of the Micro/Nanotechnology lecture in the Physics or Photonics Master's programme and the associated seminar. Practical training has not yet been provided. This gap is to be closed by expanding the programme of the lab course.
The aim of this offer is to gain initial experience with an existing lithography line in the clean room and to jointly develop a concept for how this can be used for future teaching.
Contact:
Supervisor: Dr. Thomas Siefke
Venue: Clean room of the IFK and F-Praktikum
The topic is suitable for one or two students.
-
Nonlinear Optics with a Twist
Light can carry different types of momentum, namely spin angular (SAM) and/or orbital angular momentum (OAM), where the latter describes the twisting pattern of a light wave’s phase front. Unlike SAM which is limited to just two states (left- or right-handed circular polarization), OAM can take on an unlimited number of values. This makes it an exciting tool for optical communication, where information can be encoded in many distinct states, greatly increasing data capacity. OAM beams are also highly resistant to distortions, making them useful for free-space optical communication and secure data transfer, as their phase structure is difficult to intercept. Beyond communications, OAM plays a crucial role in advanced optical technologies, including imaging, quantum computing, and light-matter interactions.
Recent research has focused on generating and controlling OAM in atomically thin materials, where strong nonlinear optical effects can efficiently shape vortex beams at the nanoscale. Layered van der Waals materials, such as transition metal dichalcogenides, provide a powerful platform for the study of nonlinear optical processes. Unlike bulk materials, these ultra-thin systems do not suffer from phase-matching limitations, allowing for highly tunable light generation across a broad spectral range. By exploiting nonlinear effects like sum frequency generation, difference frequency generation, and four-wave mixing, researchers can manipulate OAM in ways that were previously inaccessible at such small scales.
In this series of experiments, we will investigate how different layered van der Waals materials influence the OAM of nonlinear optical signals. Specifically, we will study second-harmonic generation in rhombohedrallly stacked molybdenum disulfide (3R-MoS₂) and niobium oxide diiodide (NbOI₂). Due to their distinct crystal symmetries (C₃v and C₂), these materials exhibit characteristic selection rules that govern the OAM of the generated light. First, we will characterize the nonlinear optical response and analyze the OAM quantum number. Additionally, we will introduce SAM as an extra control parameter, tuning the fundamental beam from linear to circular polarization to observe its effect on the nonlinear OAM response.
Working plan:
Weeks 1-4: introduction to SHG in NbOI2 and 3R-MoS2, building the experimental setup to study the dependence of SHG on input power, material thickness and crystal axes
Weeks 5-8: introduction to angular momentum of light, creation of vortex beams with OAM, comparison of SHG in NbOI2 and 3R-MoS2 with OAM
Weeks 9-12: comparison of SHG with linearly/circularly polarized pump (with/without SAM)
Objectives:
- Layered materials: band structure, optical properties and crystal symmetry
- Nonlinear optics: basic theory, nonlinear susceptibility tensors for SHG, selection rules
- Polarization optics (Quarter wave plate, half wave plate, phase plate)
Experimental techniques:
- Second harmonic generation (SHG)
- OAM through phase plate
- Low noise and lock-in detection
Contact:
Supervisor: Sebastian Klimmer
Venue: GUFOS, IFK (Room E002)
The topic can be worked on by one or two students. Supervision is possible in English and German
-
Photoelectron Diffraction of Crystalline Surfaces
According to de Broglie matter has not only particle but also wave character. It was shown that electrons, due to their rest mass, already exhibit wavelengths of around 1 angstrom at acceleration voltages of about 150 V, which is in the range of atomic distances in solids. Crystals therefore represent natural diffraction gratings for accelerated electrons, just as they do for X-rays of similar wavelengths. However, due to the strong inelastic interaction between electrons and atoms, the inelastic mean free path of electrons in solids ranges from less than 1 to several 100 nm which is thus considerably smaller than for X-rays. This makes electron diffraction especially suited for the investigation of crystalline surfaces and thin layers.
The aim of this projects is to understand principles of a special type of electron diffraction, namely X-ray photoelectron diffraction (XPD). This method enables an element specific analysis of the structure of a crystalline surface. For comparison, low-energy electron diffraction (LEED) will also be performed as a widely used characterization method for inorganic compounds. Students will learn how to prepare their own samples, starting from cleaning single-crystal surfaces, followed by the deposition of films via molecular beam epitaxy as well as their structural characterization by means of LEED and XPD. All preparation and analyzing steps are performed under ultrahigh vacuum (UHV) conditions.
Goals and context
- principles and application of electron diffraction in two dimensions (2D)
- concept of reciprocal space
- preparation of atomically clean single crystals and two dimensional materials
- highly-ordered ultrathin layers by molecular beam epitaxy
- vacuum technology (pumps, gauges, rest gas analysis etc.)
Methods
- UHV chambers with:
- Photoelectron spectroscopy setup (X-ray source, hemispherical analyzer)
- MCP-LEED (electron gun, phosphor screen, micro channel plates, camera)
- sputter gun and sample heater
- vacuum pumps (roughing, turbo, ion getter, and titanium pump)
- metal single crystals as sample substrates
Contact:
Supervisor: Maximilian Schaal, Dr. Felix Otto
Venue: Labs of AG Fritz (ZAF)
The topic is suitable for two students.
-
Vacuum Coating of Thin Metal Layers
Thin layers are layers with thicknesses in the micrometer and nanometer range. Their physical parameters such as electrical conductivity often deviates from that of the bulk material, allowing for altered, tailored properties and new functionalities. In addition, the material savings are often of great economic importance. Well known is the application in the field of protection against environmental conditions, e.g. against corrosion or oxidation. However, thin layers are most important in microelectronics, where almost all components are manufactured using thin-film technology. In optics, thin layers and layer stacks are used to influence the reflection and transmission behavior, but also the polarization. In particular, layer systems play a prominent role in X-ray optics.
In the internship, metallic layers are usually deposited and characterized by different methods. Concrete topics and goals, amongst others taken from current research projects, are proposed by the supervisor at the beginning of the internship, but can be discussed and adapted depending on the interests.
Learning goals and content:
- Deposition of thin metal layers by means of various coating methods (sputter coating, thermal evaporation)
- Characterization of the layer properties (e.g., composition, roughness, crystalline properties) depending on substrate properties and coating parameters (e.g. chamber pressure, residual gas composition, process times, substrate heating)
- Introduction and application of various analysis methods
- Scanning Electron Microscopy (SEM) and Energy Dispersive X-Ray Analysis (EDX)
- Scanning Tunneling or Atomic Force Microscopy (STM, AFM)
- Auger Electron Spectroscopy (AES)
- X-ray diffractometry (in cooperation with the X-ray group)
Experimental equipment:
- Sputter coating system from Oxford Instruments
- Thermal evaporation system (self-made)
- Mass Spectrometer for residual gas analysis
- Quartz layerthickness monitor
- Scanning Electron Microscope
- Atomic Force Microscope
- Scanning Tunneling Microscope
- Auger Electron Spectrometer
Contact:
Supervisor: Dr. Thomas Siefke
Venue: F-Praktikum
The topic is suitable for one or two students.
-
Polymeric Nanoparticles for Targeted Drug Delivery
The recent emergence of nanomedicine has revolutionized the therapeutic landscape and necessitated the creation of sophisticated drug delivery systems. Polymeric nanoparticles sit at the forefront of numerous promising drug delivery designs, due to their unmatched control over physiochemical properties such as size, shape, architecture, charge, and surface functionality. Hence, a precise understanding of polymeric nanoparticles preparation and characterization is essential for optimizing the drug delivery system.
We will prepare polymeric nanoparticles with various sizes and crystallinities and characterize them by size investigation and morphology. The application of the materials is not just interesting for basic research but also promising for the targeted delivery of drugs with optimized structures.
The aim of this work is to develop a comprehensive understanding of polymeric nanoparticles as drug delivery vehicles, using the various nanoparticle designs and preparation methods. If you are interested in new, future-oriented frontier materials and physico-chemical aspects and like to work experimentally, then join our international team.
Goals and context
- Creation of nanostructured polymeric materials
- Characterization and analysis of these materials
- Structure elucidation of the materials
- Structure-property relationships of materials
- Insight into current research and development fields in nanotechnology and smart-functional materials methods
Methods
- Advanced literature research
- Elaboration of nanoprecipitation methods
- Analysis of polymeric nanoparticles, using atomic force microscopy (AFM), electron microscopy (SEM, TEM), UV-Vis, dynamic light scattering (DLS), etc.
- Determination of the mechanical properties of polymeric nanoparticles
Prerequisites
- Interest in materials science, nanophysics and soft matter physics
Contact:
Person in charge: Prof. Dr. Klaus D. Jandt
Supervisor: Dr. Chuan Yin
Venue: OSIM, Löbdergraben 32
The topic is suitable for two students. Up to tow student pairs (2 x2) may work on this topic.
-
Chirped ultra-short light pulses
Chirped laser pulses have been widely applied in physics to better control the pulse duration and energy of laser pulses, and eventually to steer the interaction of light with matter. A chirp hereby refers to laser pulses with a frequency that varies over time, and where a red-chirped pulse starts with lower frequency and ends up at a high frequencies. There are several parameters that help distinguish between different chirped ultra-short light pulses, including linear, non-linear and others. – Chirped pulses are used in fiber-optic communication, for data compression as well as for cooling and manipulating atoms, among other applications.
In this project, we wish to analyze and understand the basic (Fourier-) Transformations that help imprint a chirp upon pre-defined laser pulses. Beside of the algebraic evaluation of useful Fourier integrals, this may include numerical solutions for selected pulse shapes.
Goals of the project
- Recall the 1-dim Fourier transformation of simple pulse form.
- Compared different pulses in the time and frequency domain.
- Understand how laser pulses are formed and how light is amplified.
- Understand the notion of electron vortices.
- What is the time-frequency uncertainty and how does it affect the propagation of all electro-magnetic signals ?
Prerequisites:
- basic knowledge of quantum mechanics
- programming skills (Python, Julia, C)
Contact:
Supervision: Prof. Stephan Fritzsche, Theoretisch-Physikalisches Institut
Where: Theoretisch-Physikalisches Institut & Helmholtz-Institut Jena, Frauenhoferstr. 8.
Per term, one or two students may work on the project
-
Computer experiment: Quantum tomography of photonic qubits
Quantum tomography has been widely applied in quantum computing and quantum information theory during recent years to determine the state vector (density matrix) of simple quantum systems. In contrast to a single quantum measurement, which randomly leads to one of the eigenvalues (outcomes) and eigenvectors (post-measurement states) of an observable (hermitian operator), quantum tomography aims to reconstruct the full quantum state as it is assigned to the system prior to the measurement.
To reconstruct the (initially) unknown quantum state of a system, large ensembles of identical prepared quantum systems (states) are typically required. Moreover, a tomographically com-plete set of measurements need to be chosen to determine a quantum state uniquely. Mathe-matically, this means that this set of measurement operators need to form a valid (operator) basis for the Hilbert space of the given system. In this project, we wish to analyze the polarization state of single photons as well as of photon pairs, similar as generated in the spontaneous parametric down conversion process and as applied for EPR type experiment.
This project aims to reconstruct the density matrices of single- and two-photon quantum states from a simulated measurement statistics. Different methods from linear algebra and the least-square-optimization should be explored and compared to each other.
Goals of the project
- Recall and understand the formalism of the density matrix and quantum measurements (measurement operators) in order to obtain and analyze the outcome of any (computer) experiment.
- Explore the close relation between the polarization state of photons and spin-1/2 particles (like electrons, protons, …).
- Work with the (generalized) Pauli matrices and the correlation tensor of few-qubit systems.
- Understand to role of (quantum-) tomographic methods for analyzing simple quantum gates.
- Design a program code for the reconstruction of the density matrix from the generated outcomes (measurement statistics)
- explore and determine the scaling of the required effort for n-qubit systems.
- optional extensions: What means and how can one perform quantum process tomography ?
Prerequisites:
- basic knowledge of quantum mechanics
- programming skills (Python, Julia, C)
Contact:
Supervision: Prof. Stephan Fritzsche, Theoretisch-Physikalisches Institut
Per term, one or two students may work on the project
-
Dielectronic recombination of hydrogenic ions: A key to relativistic quantum physics.
The dielectronic recombination (DR) of multiply and highly-charged ions involves the capture of an electron due to the resonant excitation of another bound electron as well as their subsequent stabilization by photon emission. The DR process has been found essential for understanding the dynamics of highly-ionized plasma in astrophysical objects, fusion reactors, and at several places elsewhere. In astrophysics, for example, DR affects the ionization balance of gas in galaxy clusters and the intergalactic medium and, hence, the formation of stars and large-scale structures in the universe.
In this project, we wish to explore and compute the DR resonance strength for the capture of electrons by (selected) hydrogenic ions with medium or high nuclear charge, Z. This requires to determine and apply solutions to the Dirac equation as they are provided by several (atomic) codes. We wish to analyze the low-lying DR spectrum for such ions and to compare our theoretical predictions with available experimental data.
Goals of the project
- Recall the treatment of one- and few-electron ions in terms of wave functions.
- Formulate the DR resonances strength by means of two-electron matrix elements.
- Describe the interaction of atoms with a weak radiation field.
- Understand and apply an existing (Julia) code in order to compute the DR strength and plasma rate coefficients.
- Optional: Compare different theoretical models and how well they compare with experiment.
Prerequisites:
- basic knowledge of quantum mechanics
- programming skills (Python, Julia, C)
Contact:
Supervision: Prof. Stephan Fritzsche, Theoretisch-Physikalisches Institut
Where: Theoretisch-Physikalisches Institut & Helmholtz-Institut Jena, Frauenhoferstr. 8.
Per term, one or two students may work on the project
-
Modern Topics in Quantum and Gravitational Theories
Possible topics within this project are:
- Entanglement and its entropy measures in quantum mechanics
- Supersymmetric quantum mechanics
- Magnetic monopoles and quantization of electric charge
- Magnetic monopoles in theoretical condensed matter physics: From the Berry phase in quantum mechanics to the field theoretical description of Weyl semimetals
- Do particles exist interpolating between a fermionic and a bosonic behaviour? Anyons and their description in terms of Chern-Simons theory.
- Hawking radiation and evaporating quantum black holes*
*basic knowledge of general relativity and quantum field theory required.
Contact
Supervision: Prof. Dr. Martin Ammon
Venue: Theoretisch-Physikalisches Institut, Fröbelstieg 1 (Abbeanum)
One or two students may work on this topic per term.
-
Navier Stokes Equation
Goals and context
Computational Fluid Dynamics (CFD) is a central part of computational physics and has been a driver for the development of modern numerical methods. It involves solving flow mechanical problems that cannot be solved analytically and are expensive to study experimentally by integrating generally nonlinear, partial differential equations.
In this project, the students will apply numerical methods to solve the Navier-Stokes-Equations for the case of an incompressible fluid to study flow within a cavity and flow around obstacles.
Methods
Timestepping schemes for integration of hyperbolic equations as well as a relaxation scheme for solving elliptic equations will be employed to solve the Navier-Stokes-Equations numerically on a staggered grid in two dimensions. The stability and convergence behavior of these schemes will be examinated.
The students will use C/C++, Python or Matlab to implement these methods.
Prerequisites
- Basic knowledge of partial differential equations
- Basic knowledge of numerical methods
- Familiarity with at least one of the suggested programming languages
Contact:
Person in charge: Prof. Dr. Bernd Brügmann
Supervision: Praveer Gollapudi
Place: Abbeanum, Fröbelstieg 1 or PAF Computerpool
Per term, one or two students may work on the topic
-
Rigorous Numerical Simulation of Quantum-Photonic Nanostructures
Goals and context
The strong coupling of light to quantum systems relies on the confinement of electromagnetic fields to sub-wavelength volumes. This can be achieved by hybrid nanophotonic quantum systems, in which photonic nanostructures support tightly confined electromagnetic resonances. Computer simulations are an essential part of this research since the fabrication of nanoscopic structures is challenging and the experimental characterization of optical fields at the few photon level with nanometer resolution is equally complicated. Therefore, reliable simulation methods are required to calculate the electromagnetic response of nanostructured matter in advance. Since we are dealing with structures in the sub-wavelength range, "rigorous" methods are needed, which solve Maxwell´s equations without any approximation. Different approaches have explored for certain classes of nanophotonic structures (micro and nano cavities, metasurfaces, nanoantennas).
Methods
The students will implement and use a rigorous numerical method (FDTD or FEM) for the solution of electrodynamic problems. They will either use one of the existing professional implementations of such methods or will be working on their own implementation in a programming language suitable for high-performance computing. The method will be used to simulate the behavior of a nanophotonic structure and to investigate the coupling to quantum systems.
Programming can be done in any language preferred by the students, but Python and Matlab are supported by existing implementations.
Prerequisites
- Basic knowledge of electrodynamics and related partial differential equations
- Basic knowledge of optics
- Basic knowledge of numerical methods
- Familiar with at least one programming language supporting numerical simulations (preferred Python or Matlab)
Contact:
Person in charge: Prof. Dr. Thomas Pertsch
Supervisor: Dr. Ángela Barreda
Place: Abbe Center of Photonics, Campus Beutenberg
Per term, one group of one or two students may work on the topic.
-
Simulating a quantum computer
Goals and Context of the project
Quantum computers promise a runtime advantage compared to their classical counterparts for certain tasks. However, currently existing quantum devices operate with moderate numbers of qubits and are prone to errors due to experimental noise and decoherence. To show that quantum computers provide an actual advantage requires outperforming the best algorithms for simulating quantum dynamics on classical computer, which led to a race between scaling up quantum hardware and improving classical simulation algorithms.
The goal of this project is to build a classical emulator of quantum circuits and to use it to simulate one of the major quantum algorithms. Also, you will explore how to model noise and errors in quantum devices. The focus of the project can be adapted depending on pace and interests of the students.
Methods
- Quantum gates, quantum circuit model, quantum algorithms
- Open system dynamics simulation through quantum channels/Lindblad mater equation
- Numerical methods for linear algebra: Sparse matrices, eigenvalue problems
- Numerical solution of ordinary differential equations, numerical integrators
- Visualization tools for numerical data
- Use of libraries for quantum circuit emulation like qiskit, qutip etc.
Instructions will be provided in Jupyter notbooks with code examples in Python. If preferred, another programming language can be used.
Prerequisites:
Solid knowledge quantum mechanics
Basic knowledge of numerical methods
Familiarity with Python programming language and use of numpy/scipy libraries (or another programming language suitable for numerical simulation such as Julia, Matlab,...)
Basic knowledge of quantum optics is useful
Contact:
Person in charge: Prof. Dr. Martin Gärttner
Supervisor: Adrian Aasen, Prof. Dr. Martin Gärttner
Place: IFTO, Abbeanum
Per term, one group of one or two students may work on the topic.
-
Wave Equation
Goals and context
- Basic concept of hyperbolic partial differential equations (PDEs) and the initial-boundary value problem (IVBP)
- Finite differencing methods for derivative approximation
- Method-of-line for time-domain PDEs with Runge-Kutta timesteps
- Numerical implementation of methods to solve multi-D PDEs
- Concepts of numerical stability and convergence
Methods
The students will solve the IBVP with the wave equation in 1+1 and 2+1 dimensions (one time dimension and one and two spatial dimensions) numerically. The project has different sequential steps:
- Wave equation and reduction to first order system
- Characteristic analysis and well-posedness
- Finite differencing approximation of derivatives and convergence
- Runge-Kutta time integrators
- Solution of IBVP withthe 1+1 wave equation and periodic boundaries using the method of lines
- Stability and convergence
- IBVP with open boundaries and Sommerfeld boundary conditions
- Wave equation with a potential: the Regge-Wheeler equation, scattering of graviational waves off a black hole and quasi-normal modes
- More spatial dimensions: the 2+1 wave equation
Students can code in their preferred language, although Python is strongly recommended (open sources, simple and optimal for visualizations).
Prerequisites
- Basic knowledge of partial differential equations
- Basic programming skills
Contact:
Person of charge: Prof. Dr. S. Bernuzzi
Supervision:
Place: Abbeanum, Fröbelstieg 1 or PAF Computerpool
Per term, one or two or three students may work on the topic.
-
Detection of Runaway Stars inside the Supernova Remnants
Contents and learning objectives
Runaway stars are young stars showing a peculiarly high velocity with respect to the host cluster or OB association. They are moving away from their birth place, while the majority of others remain in their birth cluster. The high velocity nature of runaway stars is explained by two independent mechanisms:
- Dynamical ejection scenario (DES) proposes that the stars are ejected by gravitational interaction within the dense cores of the young clusters.
- Binary supernova scenario (BSS) brings an alternative explanation that the star is ejected by its orbital velocity due to the supernova of the binary companion.
Kinematic studies and observations of the young clusters has proven that the DES is working. On the other hand, high space velocities of isolated neutron stars imply that BSS is also viable. However, the star HD37424 is the only BSS runaway star which has been proven by observations. Finding BSS runaway stars provide us with great information on the supernova, supernova remnants (SNR) and neutron stars. The type of supernova, distance, age and all dependent parameters of the supernova remnant, the mass of the progenitor star and the kick given to the neutron star can be found. Therefore, exploring BSS runaways is now an important task in astrophysics. The BSS runaways can be found inside the supernova remnants (e.g. Spaghetti Nebula). The astrometry by the Gaia Satellite gives us precise distances and transverse motion vectors of the stars. A star having a significantly higher transverse velocity w.r.t the galactic neighborhood and moving away from an SNR is a potential candidate. These candidates can be detected through public astrometry and photometry data and be confirmed by spectroscopy. The low-medium resolution spectra of LAMSOT survey will be used.
The project aims to teach students using astronomical catalogs, stellar kinematics, stellar photometry, and spectroscopy.
Tasks
- Selection of stars within a certain position and distance range.
- Calculating the peculiar transverse velocity from proper motion of the stars
- Age estimates and tracing back the stellar motion in time
- Temperature and evolutionary stage estimation from photometry
- Analysis of archival stellar spectra
Contact:
Supervisor: Dr. Baha Dincel
Location : Astrophysical Institute, Schillergäßchen 2, Jena
The tasks can be worked on in a groups of 2 students.
-
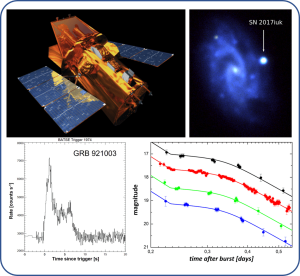
Gamma-Ray Burst Afterglows (not available in WS 24/25)
illustration of the Swift satellite (top left); host galaxy of GRB 171205A and associated GRB-SN 2017iuk (top right); light curve of GRB 921003 (bottom left); GRB-afterglow light curve of GRB 150413A (bottom right)
Foto: Sebastian SchmidlFrom the discovery of the first Gamma-Ray Burst (GRBs) in 1967, it took nearly 30 years to discover an optical transient related to a GRB, which allowed to place them at cosmologic distances. Since the 90's our knowledge of those cataclysmic events (emitted energy in gamma-rays: ~1051 - 1053 erg) has drastically expanded. We know today that these short-time gamma-ray sources (duration: a few 0.1 sec to several 100000 sec) can be found at redshifts z = 0.0085 to 9.4 (correlates to light travel time of 0.12 Gly to 13 Gly) and can be divided into two categories (long and short burst). Whereas long bursts (duration > 2 sec) are related to a special variant of type Ic supernova and short bursts (duration < 2 sec) are produced by the merger of two compact objects (preferably two neutron stars). The creation of the gamma-ray burst itself can be described within the fireball model by the collision of multiple shells traveling at high-relativistic velocities. After the burst, one can observe the afterglow of the GRB (from X-Ray to the Radio), which arises from the interaction of the shells and the interstellar material (ISM) and can be observed for several days to weeks.
The Project will focus on diffent aspects and caracteristics of the optical/NIR transients that follows the appearance of a GRB and the porperties of their host galaxies.
Tasks and Learning goals
- Reduction of photometric data in the VIS and NIR
- Analysis (photometry and astrometry) of photometric data
- Modeling of Afterglow light curves to derive the main properties of the transient(time and spectral evolution)
- search and modelling of Supernova components that can be found in the light curve
- investigating the properties of the GRB host galaxies (e.g. mass, age of the dominant stellar population, star formation rate)
- search within public archives for additonal data (data mining)
- deepening the understanding of relativistic outflows, Supernovae and the host galaxies of those events
- Observations at the TLS Tautenburg with the 2m Schmidt Telescope, if weather conditions are acceptable
Contact:
Supervision: Dr. habil Sylvio Klose; Dr. Sebastian Schmidl
Location: Thüringer Landessternwarte Tautenburg (TLS Tautenburg) and/or F-Pranktikum (please contact S. Schmidl for further informations)
Students can consider for example, to spend one day every two weeks in Tautenburg to work on the project.
-
N-Body-Simulation of Planet Dynamics
Context and goals:
In this project, mutual gravitational perturbations in systems containing stars, planets, and minor bodies are studied. Depending on the scenario and configuration, these perturbations can lead to different types of short and long-term phenomena: resonances and chaotic behavior as well as secular effects. Possible examples for specific scenarios include: capture in and release from orbital resonances; long-term stability of planetary systems; Lyapunov exponent and chaotic motion; influence of small perturbers on chaotic systems; secular perihelion drift in multi-planet systems; Kozai mechanism. For each specific problem, analytic approximations are available and can be used for comparison with the numerical results.
Methods:
A handful of numerical integrators is available, covering a set of different algorithms (Bulirsch-Stoer, Runge-Kutta, Everhart, (hybrid) symplectic) and scenarios. The integrators can be compared with respect to their precision and speed. Simulation results can then be visualized and statistically examined with self-made programs/scripts.
Contact:
Instructor: Dr. Torsten Löhne
Venue: Astrophys. Inst. and Unisternwarte, Haus 2 (Schillergässchen 3)
The complex topic can be worked on by one or 2 students. The actual tasks will be adapted.
-
Hydrostatic Models of Planet Interiors and Atmospheres
Goals and context:
Most of what we know about the internal structure of planets in the solar system ( and elsewhere) is based on observations of their external properties - and on models. Such models need to cover an extreme range of physical conditions and states, from low-pressure gas to high-pressure liquids and solids, including "exotic" material succh as liquid hydrogen. In this project, simple models of planet interiors and atmospheres are constructed and tested against more refined models and observational constraints. Possible examples for specific goals include:
- test of the limits of simple models (When do they work? When and how do they fail?)
- implementaion and test of more advanced analytic approximations of equations of state or tabulated material properties (When und where are they useful?)
- construction of more complex models (What role do internal enegy souces and the temperature gradient play?)
Methods:
Available analytic and numerical prescriptions for hydrostatic equilibrium, equations of state, and heat transfer are used to construct a new model or modify an existing one. Additional material properties and constraints are extracted from the literature. Possible languages for programming (and in most cases also visualization of the results) include C++, Wolfram Mathematica, Phyton, Mathlab.
Contact:
Instructor: Dr. Torsten Löhne
Venue: online and/or Astrophys. Inst. and Unisternwarte, Haus 2 (Schillergässchen 3)
The complex topic can be worked on by one or 2 students. The actual tasks will be adapted. Supervision is possible in English and German.